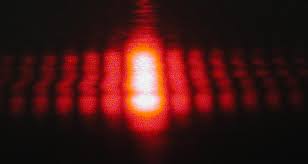
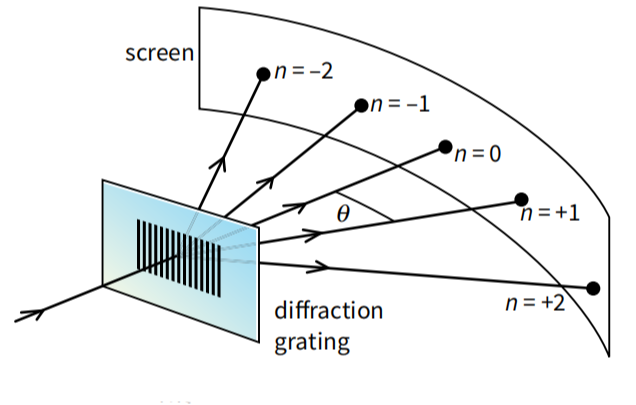
How a pattern is formed
- Diffraction: When light passes through each slit or line on the grating, it diffracts or spreads out.
- Interference: These diffracted light waves then overlap and interfere with each other.
- The interference can be constructive, where waves add together to make a bright spot, called the maxima.
- They can also be destructive, where they cancel each other out, creating a dark area.